Your Favorite Shape

Examining shapes and the geometric language of the universe.

---

What is Your Favorite Shape?
This is a far more interesting question than: what is your favorite color?, your favorite food or just about any other small talk topic.

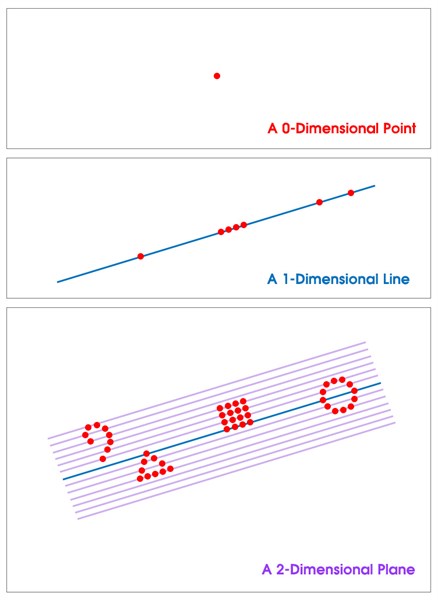
When someone asks you what your favorite shape is, your first question back to them should be: in how many dimensions?  1D, 2D, or 3D?

For me, I would say my favorite shape is the spiral. I’ve gone by the moniker @CreativeSpiral for over 20 years in various forums and for my creative business practices. But what is the dimensionality of a spiral?  A spiral is a one dimensional shape. It is a 1D line with a continuous curve to it... sometimes logarithmic, sometimes archimedean, and sometimes a more abstract spiral or other ratio based on the forces of physics, as in the "orbits" of celestial bodies (planets and star orbits are not actually perfect circles/ovals, but rather spirals).    

Most people, when asked what their favorite shape is, will give you an answer of a two dimensional shape - for instance: a circle a square a triangle or maybe something more exotic like a hexagon or octagon. These are all closed two dimensional shapes. Some others when asked this question may respond with a three-dimensional shape such as a sphere a cube, Or something more extravagant like a dodecahedron.

The Language of the Universe is Mathematics Geometry
So why is this a more interesting question to ask then what is someone’s favorite color or food? Well, dimensions are inherently built into the structure of the universe. Asking someone what their favorite shape is can open up some much more interesting discussions. Indeed dimensions are an underpinning of the very language of nature and mathematics. It is often said that mathematics is the language of the universe, but what is mathematics really? Mathematics, at its core is a way to describe operations done from a dimensional perspective.

When we add two numbers, we are talking about extending a one dimensional value. When we talk about division or multiplication, we are talking about dimensionally promoting or extruding a one dimensional value into a two dimensional shape, or turning a two dimensional area into a three dimensional shape.

If we’re talking about a one dimensional line that is five units long - i.e. the number five, and we are adding three more units, then that one dimensional line becomes eight units long. However if we take that five unit line that is one dimensional, and we say let’s multiply it by three, we are saying it’s time to dimensionally promote that one dimensional line, and extrude it into the second dimension. So we take that five unit line and multiply it by three, and now we have a rectangular shape, with a long side of five units, and it is extruded by three units on the short side. The total area of this new two dimensional shape is 15 units. 5×3. So talking about dimensions and shapes is fundamental to our understanding of nature.

Understanding Dimensions is a Key Concept to Understanding the Universe
Let’s talk a bit about one dimensional shapes. The most basic one dimensional shape is a straight line.  A one dimensional shape need not be straight though. One can imagine a one dimensional line being “bent by space-time “as an analogy. When a one dimensional line is bent it may become a curve or shaped like a spiral where it loops around an imagined zero dimensional radius point. One way to test if a shape is a certain dimension is to discuss what happens if you dimensionally promote that shape, or extrude that shape along the next dimension. If you take a straight line and promote it from one dimension to two dimensions, by extruding it to that next dimension, that line becomes a square.

Likewise if you take a one dimensional spiral shape and extruded along the next dimension, you also get a square or rectangle shape, however your square or rectangle is folded or rolled in on itself. If you unroll it, you get a similar two dimensional square or rectangle as when you extruded a straight line. Therefore we see a spiral, like a straight line, is a one dimensional shape – it just has been bent, like how gravity bends space-time. 

If you take a two dimensional shape, such as a square or a triangle, and extrude it or promote it to the next dimension, you get a three dimensional object. In the case of starting with a square, when you extruded along the next dimension, it may become a cube - which is a three dimensional shape.

Now let’s talk about a circle. A circle is a unique shape in that it can be interpreted as either a one dimensional line that curves back on itself perfectly to meet at its origin point, or you can interpret the circle shaped as being a closed to dimensional shape that is filled in. Why is this interesting? There may be some mathematical significance to the fact that a perfect circle can be represented as either one dimensional or two dimensional depending on the context.  More to come on this, and the meaning of π (pi).